Comparisons: Plurality (First-Past-The-Post) and Anti-Plurality 2

Features of Simultaneous Single-Winner Plurality (FPTP) Elections

FPTP is often used in multiple contests where there are parallel elections in multiple constituencies and one winner in each. Non-proportional outcomes are virtually inevitable from such elections. Parties with fairly uniform support across most constituencies will lose out to ones having similar overall support but with strong winning shares in many seats yet negligible amounts in others. Also, perverse outcomes where a party gains more votes yet fewer seats than another party are equally inevitable with such dissimilar geographic vote distributions. A party can even have a majority of seats despite it having a relatively small share of the overall vote or despite another party having more votes. Again, polarization beats consensus in plurality elections.

In FPTP elections, many votes are effectively 'wasted'. Votes for all losing candidates and votes for the winning candidate in excess of that required to win are votes that need not have been cast as the end result is the same. In multiple single-winner FPTP elections with several candidates competing in each constituency, the number of wasted votes may typically exceed those that are not wasted.

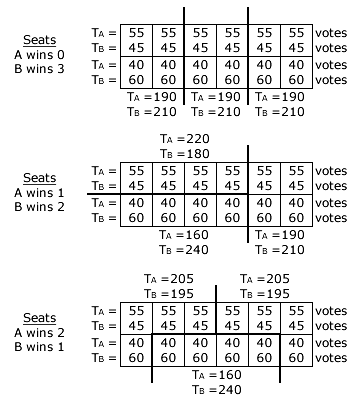
Given the generally large proportion of wasted votes, the potential for 'gerrymandering' is extensive. In the examples opposite, there are four districts in each of the three constituencies. The tally of votes cast (T) for parties A and B in each district are as shown. How many seats each party wins depends entirely on how the twelve districts are partitioned into the three FPTP constituencies; these constituency boundaries being shown as bold lines.

With CHPV, the three single-winner FPTP constituencies would be replaced with a single three-winner one; see the preceding Evaluations (Party-List) chapter. Here, the twelve districts would form one three-seat constituency with parties A and B gaining one and two seats respectively given the slightly higher overall tally for B.

However, using FPTP, constituency boundaries can be drawn to favour either party A (as in the bottom example of partitioning) or party B (as in the top example). The optimally proportional outcome is shown in the middle example. In the bottom example, party A gains two seats with an excess of 10 votes in each while the more preferred party B gains just one seat with an excess of 80 wasted votes.

Unfortunately, as in the top example, evening out these wasted excess votes across the three constituencies only distorts the result in the opposite direction; no seats for A despite it having more than one third of the vote. Unscrupulous governing parties favour plurality as it can enable them to retain power purely by manipulating or 'gerrymandering' boundaries to their own advantage.

Parties do not waste valuable resources on 'safe' or 'no-hope' constituencies where they confidently expect to retain their existing status as winner or loser respectively. They focus instead on 'marginal' ones that can be won or lost. Similarly, parties concentrate on the 'swing' voters in these marginals who could change their vote and hence the election outcome; largely ignoring committed voters. With such plurality elections, a majority of overall voters may well have no effect on an election outcome. Unsurprisingly, the very restrictive nature of voter ballots with FPTP frequently breeds alienation and often yields a poor turnout of voters and an unrepresentative result.

Properties of Anti-Plurality

Anti-plurality satisfies all those criteria that all positional voting systems comply with. Unlike plurality however, it fails the majority criterion. Indeed, the winner may not receive any first preferences at all; as in the election example on the previous page. The negative vote tallies for the four candidates are -38 for A, -40 for B, -22 for C and 0 for D. With the consensus candidate D attracting no last preferences and hence no negative votes, D easily wins this election. This contrasts markedly to the plurality election where D came last in the candidate rankings.

As their names suggest, plurality and anti-plurality are opposite extremes. All GV systems including CHPV have their own corresponding anti-versions too. The outcome of anti-plurality and anti-GV elections can also be manipulated by tactical voting and the strategic nomination of candidates. These topics and such voting systems are explored in later sections of this chapter.

Proceed to next section > Comparisons: Borda Count

Return to previous page > Comparisons: Plurality (FPTP) and Anti-Plurality 1