Comparisons: Sainte-Laguë ~ Party Cloning 1

Susceptibility of Sainte-Laguë Party-List Elections to Party Cloning

Party cloning threshold figures are introduced in the earlier Evaluations: Party Cloning section where the figures for CHPV are evaluated against those for an optimally proportional voting (OPV) system. The relevant threshold figures for the Sainte-Laguë method are derived using the same methodology as explained in this earlier section. For up to six winners, the benchmark OPV figures are repeated below left and the corresponding figures for the Sainte-Laguë method are shown below right.

To recap, the green line is the two-party map for parties A and B. The red line is the 1:1 two-way tie line for parties B₁ and B₂ on the three-party map for A, B₁ and B₂. The ranges of the tally shares for party A (t_A) that produce a different seat share for A:B (as stated in each domain) after party B clones itself into parties B₁ and B₂ are highlighted in white.

Any particular tally share threshold for party B may rise, fall or remain unchanged following its cloning attempt. Where it is a rise, party B succeeds only in harming its own chances. The black line within any white area indicates the change in the tally share threshold needed to maintain the (critical-tie) boundary between the two relevant seat share domains. It has a positive gradient for a rise in this threshold. Where it is a falling threshold, party B gains an unfair advantage over A. The black line now has a negative slope.

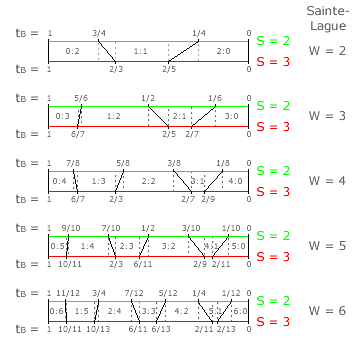
From the Sainte-Laguë threshold figures, it is clear that cloning by party B always has an effect on every seat threshold. In half of the cases, B suffers self-harm but, in the other half, it gains an unfair advantage in winning seats. The chances of cloning altering the seat share ratio between the parties increases as the share of the vote for B decreases. In this election scenario, the Sainte-Laguë method is hence readily susceptible to strategic nominations through party cloning.

The Sainte-Laguë method with its very high degree of optimality is similar to the Hare Quota (OPV) where its optimality is perfect. Both are also significantly vulnerable to party-cloning strategic nominations. Comparing the two most popular highest averages approaches, the Sainte-Laguë method has a higher optimality than the D'Hondt approach but d'Hondt is not as susceptible to party cloning as is Sainte-Laguë. As seen earlier with the two most popular largest remainder methods, this same trade-off exists between the Hare and Droop Quotas. The Hare Quota method has the better optimality while the Droop Quota method is much less susceptible to party cloning.

Proceed to next page > Comparisons: Sainte-Laguë ~ Party Cloning 2

Return to previous page > Comparisons: Sainte-Laguë ~ Optimality 2