Evaluations: Clones, Teaming and Independence Criteria 4

Tied Voter Preferences and Teaming

Before considering teaming effects in more detail, the reason why tied voter preferences are not permitted can now be explained. Although voters may genuinely have no preference for one particular candidate over another, allowing them to award the same rank to such candidates does adversely affect the properties of CHPV. The critical feature that the weighting of any rank is always larger than the sum of all the weightings of lower rank ceases to be true. Tied voter rankings also generate additional teaming opportunities as the following trivial two-voter three-candidate CHPV election demonstrates.

The candidate-listing ranked ballots for each of the two voters is shown opposite and the resultant tallies for the three candidates (using weights of 4, 2 and 1) are given below. The outcome is a tie for first place between A and C with B the clear loser.

• T_A = 4 + 1 = 5
• T_B = 2 + 2 = 4
• T_C = 1 + 4 = 5

If tied rankings were permitted, either A or C could have introduced a clone of its self to 'bury' the other and so become the sole winner. Suppose that C did do this and that C₂ is the clone of C₁. This scenario is reflected in the two ranked ballots shown below. Notice that the A-supporting voter 1 cannot distinguish between the two C clones so awards third place to both but that C has added C₂ specifically to push A down into fourth place. With four ranks, the weightings are now 8, 4, 2 and 1. However, there are two competing options for handling such tied preferences. The first one is to employ the average value of the tied ranks. In this example, the clones are in joint third place so the average of the weightings for third and fourth positions is (2+1)/2 or 1.5. This maintains that the same total of points (15 here) is awarded by every voter. The resultant candidate tallies (for both handling methods) are given below. By teaming, C succeeds in gaining an unfair victory over A.

• T_A = 8 + 1 = 9
• T_B = 4 + 2 = 6
• T_C1 = 1.5 + 8 = 9.5 or alternatively T_C1 = 2 + 8 = 10
• T_C2 = 1.5 + 4 = 5.5 or alternatively T_C2 = 2 + 4 = 6

The alternative way to handle tied preferences it to simply award the stated rank to each tied candidate. Here, the two clones are each given 2 points as this weighting is specified for third place. Voter 1 now awards 16 points in total unlike voter 2 but the extra point is shared by the cloned C candidates that voter 1 likes least. Through teaming, C now wins by an even greater margin over A.

Sticking with awarding the stated rank to tied preferences, voter 2 could have given equal first place to the two clones instead of first and second as shown in the ranked ballot below left. Candidate A is still pushed into fourth place either way. The updated candidate tallies below highlight the exaggerated outcome where both C clones now beat A.

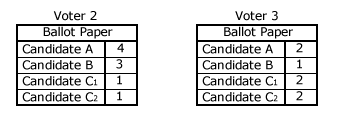
• T_A = 8 + 1 = 9
• T_B = 4 + 2 = 6
• T_C1 = 2 + 8 = 10 or alternatively T_C1 = 1.5 + 6 = 7.5
• T_C2 = 2 + 8 = 10 or alternatively T_C2 = 1.5 + 6 = 7.5

The averaging method of handling tied preferences however prevents such an attempt at cheating through teaming. As the two clones equally share the value of first and second weightings (6 points each), candidate A now beats both of them so such attempts fails here.

Although this method of dealing with ties appears to be the preferable option, it has its own unique disadvantage. Candidate tallies are always 100% accurate as rounding is never required or permitted; see the Description: Counting section. This ensures that false ties and hence false outcomes are never generated. If tied voter preferences were allowed, then rounding could be necessary in practical elections where decimal or binary arithmetic is used for the tallies. For the ranked ballot shown above right where three candidates tie for second place, the tally contribution from voter 3 to each tied candidate is (4 + 2 + 1)/3 = 7/3 = 2.333.... As the divisor is not a power of two, rounding is unavoidable when such tallies are expressed in decimal or binary form.

Hence, tied voter preferences on ranked CHPV ballots are strictly forbidden as neither tie-handling method is satisfactory due to teaming, rounding is never acceptable and key properties of the voting system need to be maintained.

After one or more preferences have been expressed by a voter, truncation of further preferences is however permitted. Therefore, voters are not required to award any points to candidates that they strongly disapprove of or who are engaged in teaming attempts. Truncation is an effective punishment against teaming. Also, voters may truncate their ballots when the rump candidates remaining to be listed are viewed with ambivalence, are indistinguishable from each other or both. By awarding zero points to all unexpressed preferences, truncation effectively ties these preferences without causing any adverse system effects.

Proceed to next page > Evaluations: Clones & Teaming 5

Return to previous page > Evaluations: Clones & Teaming 3