Mathematical Proofs: Geometric Voting

Proof CG7: Common Ratio for Balance between Vote Splitting and Teaming

Scenario: The election is conducted using GV (any r) and its standard weightings are used here to determine the tally (T) for each candidate on the ballot. The weighting of the first preference (w₁) is a and the number of voters casting valid ballots is V. The clone set is A and it nominates two clones in total. On its forward slate, A₁ is the superior fraternal clone and A₂ is the inferior one. The reverse slate therefore lists A₂ ahead of A₁. All voters rank the clone candidates in either forward or reverse order. The proportions of voters ranking candidates in forward or reverse order are given below.

• p_F = Of those preferring A first, the proportion who rank the clone set in forward slate order
• p_R = Of those preferring A first, the proportion who rank the clone set in reverse slate order
• q_F = Of those not preferring A first, the proportion who rank the clone set in forward slate order
• q_R = Of those not preferring A first, the proportion who rank the clone set in reverse slate order

Hence, for those preferring A first, p_F + p_R = 1 and, for those preferring B first, q_F + q_R = 1. Where all first preference A voters rank candidates in compliance with the forward slate p_F = 1 and p_R = 0. Where they vote consistently for the reverse slate p_R = 1 and p_F = 0. If no slate is issued and all clones are identical rather than fraternal, then p_F = 1/2 and p_R = 1/2. The same holds for first preference B voters except that the proportion p is now replaced by the proportion q.

For the clone set, there is no point in teaming if it would win anyway with just one candidate A. Therefore, its easiest opportunity to win unfairly through teaming is when it is tied with all the other competing candidates (just B here). This pre-cloning critical tie between both candidates (T_A = T_B) is where each has one half of first preferences and one half of second preferences. After nominating its two clones, the profile for this election scenario is given below; using the standard notation and formats,

• p_FV/2: A₁,A₂,B.
• p_RV/2: A₂,A₁,B.
• q_FV/2: B,A₁,A₂.
• q_RV/2: B,A₂,A₁.

Consider the tally ratio T_A/T_B where A may be either A₁ or A₂ as appropriate. If this ratio is greater than one, then A beats B through teaming; while if less than one, B beats A via vote splitting. If the ratio equals one, then the original critical tie is maintained. For all combinations of p, q and r, the tallies for A₁ and A₂ are derived below.

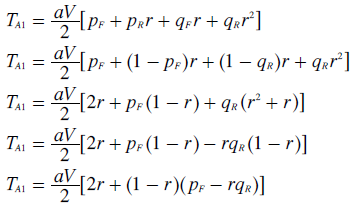
Further, the tally for B is derived below.

Hence, the two tally ratios that determine who wins and by what factor are given below.

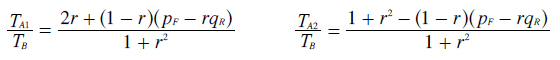
Prior to any slate being issued, the A clones are identical. Here, p_F and q_R are both equal to one half. The tally ratio for the superior fraternal clone is simplified below for this specific vote splitting situation (represented by dot 1 on the cloning map in the main text).

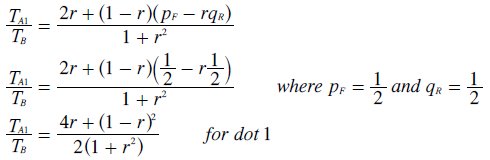
Clone set A then issues its slate and all its first preference supporters adhere to it (p_F = 1). Before first preference B supporters respond by reversing this slate, q_R remains 1/2. The tally ratio for the superior fraternal clone is again simplified below for this now teaming situation (represented by dot 3 on the cloning map in the main text).

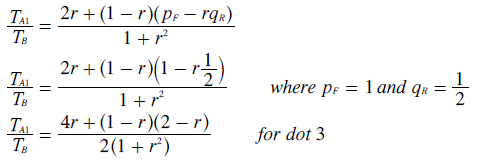
What value of the common ratio (r) achieves a balance between the magnitude of vote splitting in the case of identical cloning and the magnitude of teaming in the case of fraternal cloning? The magnitude of vote splitting is simply defined here as 1 - T_A/T_B since it increases linearly from zero as the tally ratio declines below one. Similarly, the magnitude of teaming is defined as T_A/T_B - 1 since it increases from zero as the tally ratio rises above one. There is only one value of the common ratio that generates equal magnitudes. This value of r is calculated below by first simplifying the equation for this balance and then using the standard mathematical formula for solving a quadratic equation.

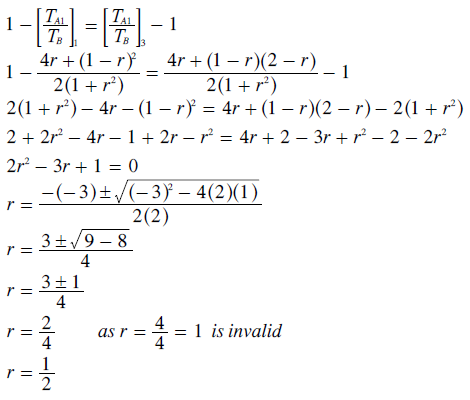
• Therefore, CHPV is the only GV variant that achieves an equal balance between vote splitting and teaming.

Return to main text > Comparisons: Geometric Voting 8

Refer to > Mathematical Proofs: Table of Contents