Mathematical Proofs: General Criteria
Proof EG2: GV (any r) fails the Condorcet Loser Criterion

Where one candidate in a particular election loses to each and every other candidate in a pairwise comparison, then this candidate is called the Condorcet Loser. If the voting system ensures that the winning candidate is never the Condorcet Loser (if there is one) irrespective of the election profile or voter input, then the Condorcet Loser criterion is satisfied.
To prove that GV with any valid common ratio (0 ≤ r < 1) fails to satisfy this criterion, only one example scenario is required; see table opposite. If the Condorcet Loser succeeds in being ranked top in this GV (any r) election, then the voting system fails to ensure that the Condorcet Loser never wins and therefore this criterion is not satisfied.
In a pairwise comparison of the ballots cast by the V voters, the fraction 1/2 + 2α of these ballots prefer candidate A to C (the type 1 and 2 ballots) but the fraction 1/2 - 2α prefer C to A (the type 3 and 4 ballots). Similarly, candidate B is preferred to C by the same +4α margin. Hence, as one candidate is defeated by all the other (two) candidates in the pairwise comparisons, there is a Condorcet Loser here and it is candidate C.
To prove that GV (any r) fails to satisfy the Condorcet Loser criterion, this example scenario only needs to demonstrate that candidate C always wins whatever the common ratio employed. The tally (TA) for candidate A and the tally (TB) for B are always equal as these two tied candidates have the same number of preferences at each rank position. The tally (TC) for candidate C must always exceed the one for A or B if C is to win this GV (any r) election.
The three candidate tallies are determined below from the data contained in the above table. Each tally is then divided by the total number of ballots (V) to yield the average tally contribution per voter for that candidate. For this proof, the fraction α tends to zero (α → 0); namely one vote in an unlimited number of votes (V → ∞).

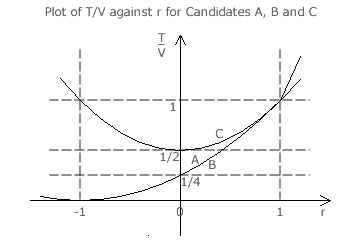
The average tally contribution (T/V) for each of the three candidates is plotted on the graph opposite as a function of the common ratio (r). For this plot, the fraction α is treated as zero. By inspection, the T/V curve for candidate C appears to approach the one for A or B when the common ratio tends towards one (within the valid range for r). For particular values of α, these two curves may in fact cross at two points, touch at one point or not even touch at all.
To accurately determine whether any valid value of r results in C losing the election, the average tally contribution for C is directly compared to that for A or B (TA/B/V). This comparison and the resultant quadratic equation are given below. The standard mathematical formula for solving a quadratic equation is then employed to derive the two values for r (if they exist) where C is on the verge of losing.
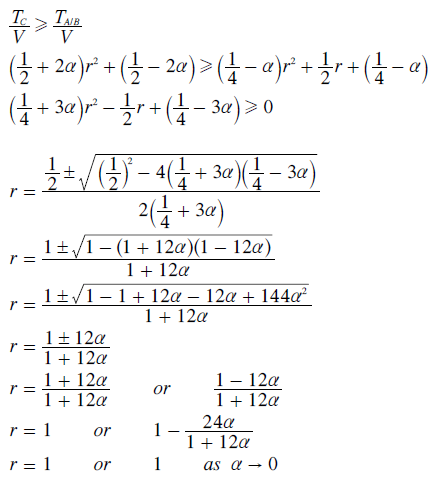
As α tends to 0, then the second value of r tends to its first value that is fixed at 1. Hence, the two T/V curves in the above graph do touch but do not cross each other. The single point at which they touch is when r is 1 and this is an invalid value for r. Therefore, for all valid values of r, the tally for C exceeds that for either A or B. So the Condorcet Loser beats both the tied candidates A and B and wins this GV election whatever the common ratio employed.
- Therefore, GV (any r) fails the Condorcet Loser criterion.
Return to main text > Evaluations: General Criteria 3
Refer to > Mathematical Proofs: Table of Contents
