Mathematical Proofs: Majority and Related Criteria

Proof EM1: Majority Threshold as a function of Common Ratio

Scenario: Candidate A has at least one half of all the first preferences; the proportion of all such ballots cast being m (where 1/2 ≤ m ≤ 1). Candidate B has all the remaining first preferences; this proportion therefore being 1 - m. Candidate B also has the second preference of every voter that ranked A as their first preference; the proportion hence being m. This scenario provides B with the best opportunity to beat A despite A having more first preferences than B.

The worst case for A (and the best for B) is extended when those voters who awarded B their first preference truncate their ballot before ranking A so awarding A a zero weighting.

Using standard notation and formats, the profile for this first election scenario is given below.

•   m : A,B,..........
• 1-m: B,..........

Alternatively, in an unlimited field of candidates (N → ∞), instead of truncation these first preference B voters could award their lowest preference to A as its weighting is vanishingly small (w_N → 0).

Using standard notation and formats, the profile for this second election scenario is given below.

•   m : A,B,**********.
• 1-m: B,**********,A.

The standard GV weightings are used here to determine the tallies for candidates A and B. In both scenarios, candidate A receives zero weightings from B supporters. The tallies for the other candidates are irrelevant as none of them can possibly beat B. For candidate A to avoid being beaten by B, the tally for A (T_A) must be greater than or equal to the tally for B (T_B). By evaluating these tallies, the required majority of first preferences (m) as a function of the common ratio (r) can be derived; as shown below.

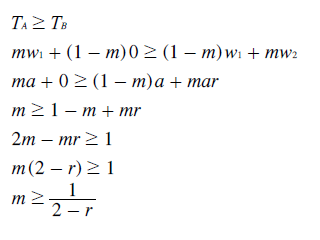
The majority threshold is therefore m = 1/(2 - r) and values of m exceeding this threshold guarantee victory to candidate A.

Return to main text > Evaluations: Majority Criteria 1

Refer to > Mathematical Proofs: Table of Contents