Mathematical Proofs: Teaming Thresholds

Proof ET5: GV Teaming Effect and Success Thresholds for Clone Set versus One Candidate

Scenario: The election is conducted using GV and its standard weightings are used here to determine the tally (T) for each of the candidates on the ballot. The weighting of the first preference is set at one (w₁ = a = 1). On the forward slate issued by clone set A, its clones are listed from A₁ to A_K. The reverse slate therefore lists these clones from A_K to A₁ in exactly the reverse sequence. Only the one candidate B has been nominated to oppose the clone set A in this non-tied election scenario. All voters rank the clone candidates in either forward or reverse order.

• p_F = Of those preferring A first, the proportion who rank the clone set in forward slate order
• p_R = Of those preferring A first, the proportion who rank the clone set in reverse slate order
• q_F = Of those not preferring A first, the proportion who rank the clone set in forward slate order
• q_R = Of those not preferring A first, the proportion who rank the clone set in reverse slate order

Hence, for those voters (V_A) awarding a first preference to A, p_F + p_R = 1 and, for those voters (V_B) awarding a first preference to B, q_F + q_R = 1. Where all the V_A voters rank candidates in strict compliance with the forward slate, p_F = 1 and p_R = 0. Where they vote consistently for the reverse slate, p_R = 1 and p_F = 0. If no slate is issued and all the clones are identical rather than fraternal, then p_F = 1/2 and p_R = 1/2. The same holds for all the V_B voters except that the proportion p is now replaced by the proportion q.

Prior to cloning, there are only two candidates but they are no longer constrained to tie (V_A ≠ V_B) so either A or B may be in the lead by any or no margin. After cloning, the number of candidates N is taken as large (essentially unlimited) so that the weighting of the two lowest preferences are negligible (effectively w_N-1 = w_N = 0). Using the standard GV notation and formats, the profile for this election scenario once party A has nominated its K clones is given below.

• p_FV_A: A₁,~~~~~~~~,A_K,B.
• p_RV_A: A_K,~~~~~~~~,A₁,B.
• q_FV_B: B,A₁,~~~~~~~~,A_K.
• q_RV_B: B,A_K,~~~~~~~~,A₁.

For a given election, the number of V_A and V_B voters is not changed by the act of cloning. However, cloning by A is intended to alter the tally for A (T_A) and for B (T_B) such that the share of the tally for A increases at the expense of B. It is therefore important to establish the boundary condition between A winning and losing both before and after cloning. In other words, the critical tie between T_A and T_B must be determined for both cases.

Prior to cloning, only V_A and V_B influence who wins the election. For this initial scenario, the common ratio (r) is not a factor in the critical tie T_A0 = T_B0; as demonstrated below.

After cloning with A₁ as the superior fraternal clone, the common ratio (r) and the relevant p and q proportions of V_A and V_B respectively all now influence the critical tie T_A1 = T_B; see below.

For a given election, V_A, V_B and r are fixed. By rearranging the above equation as shown below, the required threshold relationship between the p and q proportions for the cloning to remain unsuccessful is derived.

This equation defines the teaming success threshold. On the side of the threshold where T_A1 is greater than T_B, candidate A₁ beats B. It is important to note that the teaming success threshold equates to a straight-line relationship between p and q when plotted on the appropriate cloning map.

After cloning with A_K as the superior fraternal clone instead of A₁, the critical tie T_AK = T_B condition and its own teaming success threshold are similarly derived. They are stated below.

In the four previous proofs (ET1 to ET4), candidate A is initially tied with one or more other candidates. This means that any teaming immediately gives A victory and that any vote splitting instantly yields defeat for A. In other words, the critical threshold for teaming to occur or not - the teaming effect threshold - is identical to the critical teaming success threshold for winning or losing.

In this election scenario, A is generally not initially tied with B. This now means that although some degree of teaming or vote splitting usually occurs, it is not necessarily enough to alter the election outcome. Hence, in this scenario, the teaming effect threshold and the teaming success threshold are no longer one and the same teaming threshold. They are no longer co-incident lines on a cloning map but are now separate and independent thresholds.

The teaming success thresholds for A₁ and A_K are already established; see above. The pair of teaming effect thresholds now need to be derived. Before cloning, the tallies for A and B are equal when V_A/V_B = 1. After cloning, the two tallies are still equal when V_A/V_B = (1 - rq_F)/p_F for A₁ or when V_A/V_B = (1 - rq_R)/p_R for A_K. See above for the derivation of these equations. Therefore, for cloning to have no effect on the equality of the tallies, these pre- and post-cloning conditions must be identical. The equation for the teaming effect threshold for A₁ as the superior fraternal clone is derived below.

After cloning with A_K as the superior fraternal clone instead, its own teaming effect threshold is similarly derived. It is stated below. Again, note that each teaming effect threshold also equates to a straight-line relationship between p and q when plotted on a cloning map.

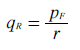
Notice that the teaming effect threshold here is identical to the teaming threshold for the election scenario in proof ET1. The only difference is that in the ET1 scenario V_A = V_B while in this scenario there is no such constraint. In the initially tied scenario, the threshold for either teaming or vote splitting to occur and the threshold for it to alter the outcome (by breaking the tie) are co-incident. Hence, a teaming threshold is simultaneously a teaming effect and success threshold.

For this general scenario where V_A and V_B are independent variables, there is no combined teaming threshold but two separate and independent ones; namely, the teaming effect threshold and the teaming success threshold. To summarise, the equations for these thresholds are restated below.

The two teaming effect threshold equations are:

• q_F = p_R/r for A₁ as the superior fraternal clone.
• q_R = p_F/r for A_K as the superior fraternal clone.

The two teaming success threshold equations are:

• q_F = [1 - p_F(V_A/V_B)]/r for A₁ as the superior fraternal clone.
• q_R = [1 - p_R(V_A/V_B)]/r for A_K as the superior fraternal clone.

These four thresholds may now be plotted on a relevant M = 1 cloning map for GV elections with a common ratio of r and specified values of the ratio V_A/V_B. Return to the main text for examples.

Return to main text > Evaluations: Teaming Thresholds 6

Refer to > Mathematical Proofs: Table of Contents