Evaluations: General Criteria 2

Pareto Condition

In the context of whether a voting system is fair or not, the Pareto condition asserts that if each and every voter prefers candidate X over an alternative candidate Y then the election outcome must reflect this unanimity and also have X ranked ahead of Y.

In the example opposite, there are three candidates standing for election. The number of different ways to complete a full ranked ballot is N factorial (N!). With the number of candidates here equal to three (N = 3), N! = 3 x 2 x 1 = 6.

Ballots types 1, 2 and 3 (as listed in the table) all rank A higher than B. The other three types (not listed) rank B above A. If all voters submitted type 1, 2 or 3 ranked ballots, then the Pareto condition requires A to be ranked ahead of B. In common with all positional voting systems, GV/CHPV would award a higher weighting to A than to B for every voter and therefore the candidate tally for A must be greater than that for B.

GV/CHPV therefore satisfies the Pareto condition.

Resolvability Criterion

To satisfy the resolvability criterion, it must be possible to resolve any tie between two or more top-ranked candidates by the addition of the ballot from one voter so that only one winning candidate is chosen.

Consider the worst case where all the candidates have identical tallies and are hence perfectly tied. For an untruncated ballot, each GV/CHPV preference is unique and worth more than the next lower one; except when the common ratio is zero (r = 0). Generally therefore, when this sole ballot is added to the count, all the ties are broken simultaneously and this individual ranking becomes the collective ranking. For the unique exception when r = 0, the tied candidate that receives the first preference - and the only one of any worth - from the additional voter is again chosen as the single winner.

GV/CHPV hence satisfies the resolvability criterion.

An alternative definition of this criterion requires that the chances of a tie occurring should tend to zero as the number of voters tends to infinity. As rounding in CHPV is neither necessary nor permitted, all candidate tallies are exact values and so only genuine ties occur. They are indeed increasing unlikely to occur as the number of voters rises without limit. [Note that the tie lines on multi-party maps that form domain boundaries are infinitesimally thin for this very reason; see later.]

Reversal Symmetry Criterion

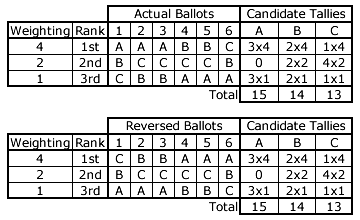
To satisfy the reversal symmetry criterion, it must not be possible for a uniquely winning candidate to still win when all the strictly ranked preferences on each ballot are reversed in rank order. Consider an example CHPV election with six voters and three candidates A, B and C; see upper table opposite. Candidate A wins with a tally of 15, B is second with 14 and C is last with 13.

Next consider the case where each of the six ballots has the order of the three preferences strictly reversed; see lower table opposite. Again, A, B and C have tallies of 15, 14 and 13 respectively. So, not only has the collective ranking not been reversed, the outcome here is identical to the actual election result. Candidate A has still won this CHPV election despite the ballots being reversed. If this same election example is reworked with any other common ratio in the range 0 ≤ r < 1, then A is still the winning candidate.

GV/CHPV hence fails to satisfy the reversal symmetry criterion.

Proceed to next page > Evaluations: General Criteria 3

Return to previous page > Evaluations: General Criteria 1